Professor Gabriel Lord

I am interested in developing new numerical methods for the numerical simulation of stochastic DEs. I have worked primarily on SPDEs but am also interested in other types of evolution equations.
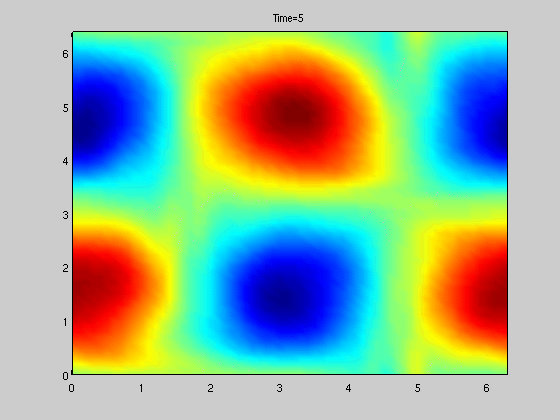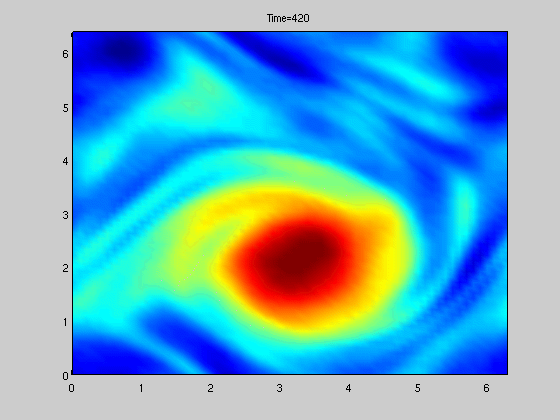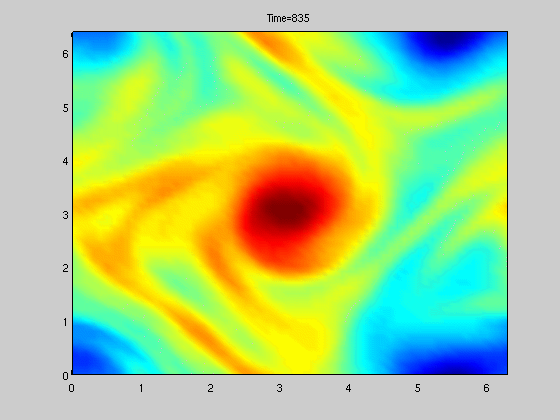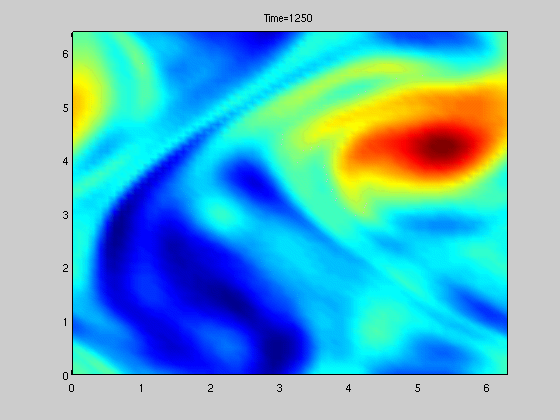
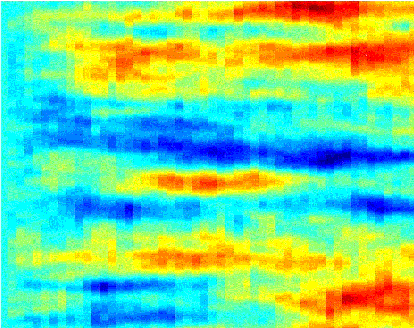
Figure to the right shows solution of a stochastically forced SPDE related to vorticity.
PhD Projects available

Accurate and efficient simulation of flow in heterogeneous porous media remains a challenging problem. The systems model potential ground water contamination, underground reservoirs, subsurface storage. In order to quantify the uncertainty in computations efficient methods are required.
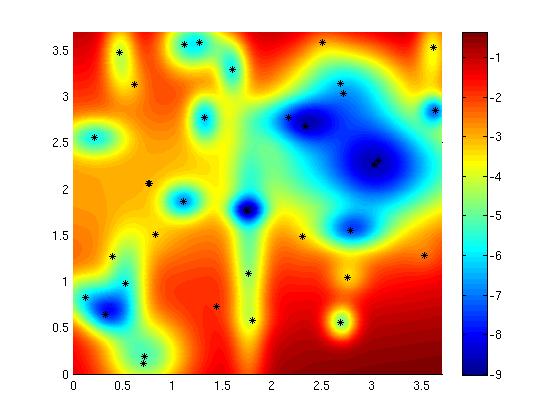
Figure shows solution of advection-reaction-diffusion equation through the SPE10 Model of a heterogeneous reservoir.
PhD Projects available

I have had a long standing interest in computational neuroscience and role of noise. Computational models are being increasingly used to gain insight into the behaviour and information processing abilities of neurons. I am interested in models of single neurons, coupled neuron dynamics as well as neural field models. More recently I have been working with experimentalists looking at the movement and interaction of vesicules and reaction with snap25 and syntaxin.
PhD Projects available

I am interested in the interaction of numerical computations and mathematical analysis and how good numerical approximations may give insights. This has included work on epsilon-entropy, global attractors and more recently the p-Laplacian.

Work on buckling cylinders in primarily driven by the need for light strong structures (such as silos, rockets, aircraft) and the desire to understand how these structures fail. As anyone who has crushed a can knows - cylinders are very strong but then buckle suddenly with a great release of energy. This work was onr of the first to look at snaking bifurcation diagrams. We also characterized the mountain pass solutions.